Lab 5 - Projections in ArcGIS

The process of map projection transforms the 3D world into a 2D model. It allows people to analyze the details on a 2D surface rather than a 3D globe because it can allow easier measurements. There are countless number of projections because they are all based on mathematics and coordinate systems, and these projections are then categorized into different groups including Conformal, Equidistant, and Equal Area. In fact, for every map projection, there will definitely be some sorts of distortions in terms of area, shape, distance, scale and direction, etc. Nevertheless, in different projections, some of the above properties are preserved and create benefits for different functions of the projections.

The Conformal projections as shown in the figures above, Stereographic and Mercator, show that this category preserves local shapes and angles. As we can see, the parallels and meridians intersect at 90 degrees angles. Therefore this type of projection is good for navigation including sailing. Since the angles do not shift while taking a bearing in certain directions. Nevertheless, Conformal projections are distorted in other ways to make it disadvantaged in certain areas. For example, shapes are not preserved within larger regions and that areas are not proportionate to the areas on Earth. When we refer to the Conformal projections figure above, we can see that Greenland in both conformal projections are elongated vertically and horizontally, for Mercator and Stereographic respectively. On the other hand, Antarctica in the Mercator projection appears to be much larger than the Antarctica in the Stereographic projection.

On the other hand, Equal Area projections preserve the area because the areas maintain proportional relationship with the Earth's. We can see from the two Equal Area projections above (Bonne and Mollweide) that the parallels and meridians are not like those in the Conformal projections, instead, they do not meet at 90 degree angles. Although the area is preserved, angles, shapes and scale are distorted. And we can see from both of the Equal Area projections above that the shapes of the regions along the margins are distorted the most, rather, the areas in the middle seem less distorted. This kind of projection is good for analyses interested in calculating areas where shape is not important.

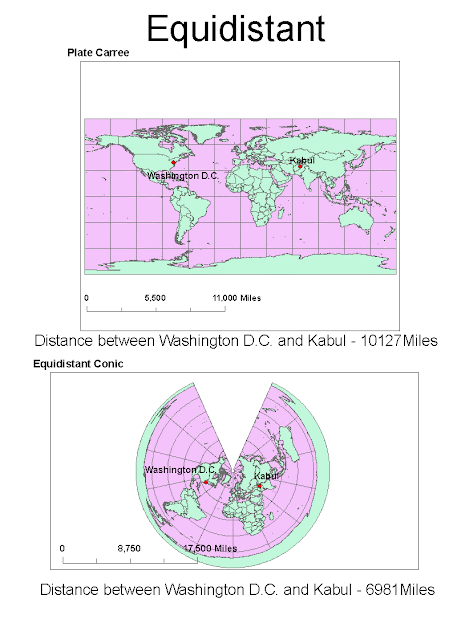
Lastly, Equidistant projections preserve distances from the center point of the map outward. Since it is impossible for any projections to preserve all the distances, therefore distances are actually maintained at a few points only. Moreover, the scale at different areas of the projection would be different. The scale of other points would be sacrificed in order to preserve the distance between points relevant for the projection. Apart from these limitations, this kind of projection also distorts the area and shape. As we can see from the two Equidistant projection maps above (Plate Carree and Equidistant Conic), that Plate Carree projection stretches the shapes of the regions sideways and the shape of Antarctica in the Equidistant Conic projection is heavily distorted.